Graphs & Charts (mostly about relationships)

I'm a fan of graphs and charts. In addition to telling a clear story behind vast quantities of seemingly impenetrable data (check out any one of Hans Rosling's TED talks or NYT's Interactive Dataviz) I like seeing them used in clever ways, especially when they (accurately!) express ideas which are strictly non-mathematical.  The webcomic indexed is based around this concept. 

Here are a few of my favorites examples of the more tongue-in-cheek kind. 

"Fluctuations of three intensities over time" (lust, infatuation & attraction) has four graphs (see the others here). It's amazing how much just a few quick colored lines can convey. Do you agree with the artist? What would the graph of your current (or former) relationship/s look like?

Kurt Vonnegut is one of my favorite authors and I've only ever read one of his books. (Cat's Cradle) Note to self: read the rest of his bibliography. Here he talks about The Shape of a Story and generalizes all stories ever told into a few simple line graphs.

Video of K.V.'s short talk on the simple shapes of stories:

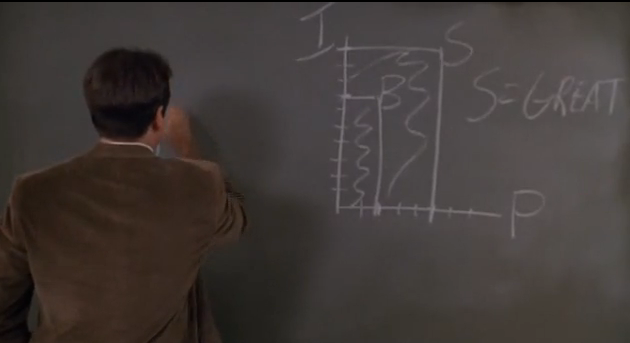
Dead Poet's Society has many great scenes, one of which involved graphing the formula P*I = G (perfection x Importance = Greatness). The greatness of the poem, then, is simply the area of the rectangle drawn out when one plots the perfection vs greatness on a plane.  This sounds like something current metric-obsessed ed reformers would eat up with a spoon. Of course it completely strips poetry of any humanity (and this meaning) and I'll be that's something else current metric-obsessed ed reformers would eat up with a spoon, too.

The scene where poetry is mesaured -- "Rip it out!" 

Arthur Mattuck is one of my favorite MIT Lecturers.  He is funny and engaging, but beyond that he makes very clever use of metaphors to help illuminate subtle and seemingly complicated ideas. Here he is using the love between George Costanza and his wife Susan from Seinfeld to model & interpret a system of linear differential equations with complex eigenvalues.

The relevant part of the lecture starts here. The full course is here.

     "And we are going to consider x is modeling Susan's love for George. And George's love for Susan will be y. Now, I don't mean the absolute love. If x and y are zero, I don't mean that they don't love each other. I just mean that that is the equilibrium value of the love. Everything else is measured as departures from that. So (0, 0) represents the normal amount of love, if love is measured... I don't know what love units are. Hearts, I guess. This is a normal equation... and this is a neurotic equation." 

Suggestions for Mathematical Exposition in Video & Writing

It's important that students learn how to solve problems and clearly communicate the thought process behind their solutions to others, usually through handwritten or typeset mathematical exposition, maybe with a few figures here and there to highlight relevant geometric aspects of the solution. Since I teach entirely online (I'm never in the classroom with my students) I feel that precise communication of ideas and solution methods are particularly important. There are often many paths to the correct answer in AP Calc, Linear Algebra, Multivariable Calc and Diffeqs. I want to know what insights and methods my students used to arrive at their answers, correct or otherwise. 

I took a relatively simple problem from differential equations 

y' = (3x^2-e^x)/(2y-5), y(0) = 1

and created a video explaining how to solve it, without me writing out every single step as I went along because I wanted to include some quick highlights during the solving itself and follow the solution with some qualitative dynamic exploration of the slope field and initial conditions using various free software such as Geogebra, dfield, and winplot.

After that, I wrote up a LaTeX document (stored on http://www.sharelatex.com)  and made a video describing some basic style & exposition guidelines for writing up one's HW or Tests in LaTeX. The same guidelines apply if writing solutions by hand.

Below is the raw LaTeX code and here is the document in PDF form.

% STYLE SUGGESTIONS
%
% When showing steps in compuations, line up equal signs vertically, one per line.
% Indent your code to make it more readable (and editable).
% Show relevant (calculus & diffeq) steps. 
% Interpret solution and steps in context of the problem & class. 
% Communicate your thought process so the reader does not have to assume or guess at what you did. 
%


\documentclass{article}


% Packages
\usepackage{amsmath, amssymb}   % math formatting & symbols
\usepackage{graphicx}           % insert graphics
\usepackage{eulervm, bookman}   % fonts for math & symbols
%\usepackage{fullpage}          % fullpage margins


\begin{document}
% Title 
\title{Seperable Differential Equation with Initial Value Example}
\author{Joe DiNoto}
\maketitle


\begin{enumerate}
    \item % 1 
        Given differential equation $y' = \dfrac{3x^2-e^x}{2y-5}$ and initial value $y(0)=1$
        \begin{enumerate}
            \item % 1a
                Solve the IVP explicitly, ($y$ as a function of $x$).
                The equation is seperable. Separate the variable and integrate. 
                \begin{align*}
                    \frac{dy}{dx}   & =\frac{3x^2-e^x}{2y-5}\\
                    \int 2y -5 \ dy & = \int 3x^2 -e^x \ dx \\
                    y^2 -5y +c_1    & = x^3 -e^x +c_2 \\
                    y^2 -5y         & = x^3 -e^x +C 
                \end{align*}    
                Combine the constants $c_2-c_1=C$. Now use the intial conditions to solve for $C$.
                \begin{align*}
                    (1)^2-5(1)  & = (0)^3-e^{(0)}+C\\
                                & \therefore \\
                            C   & = -3
                \end{align*}
                We now want to find express $y$ as a function of $x$. We can do this by noting that we ultimately have a quadratic equation in $y$. Use the quadratic formula to solve it for $y$. 
                \begin{align*}
                    y^2-4y -(x^3-e^x-3)     & = 0 \\
                    y                       & = \frac{-(-5) \pm \sqrt{(-5)^2-4(1)(-(x^3-e^x-3))}}{2(1)}\\
                    y                       & = \frac{5}{2} \pm \sqrt{13/4 + x^3-e^x}
                \end{align*}
                Again we use the intial conditions to determine if we should use the positive, negative, or both parts of the square root. 
                \begin{align*}
                    1   & = 5/2 \pm \sqrt{13/4 + (0)^3 -e^{(0)}} \\
                    1   & = 5/2 \pm 3/2 \\
                        & \therefore \\
                    y   & = \frac{5}{2} - \sqrt{13/4 + x^3-e^x}
                \end{align*}
                Because $5/2-3/2 = 1$ and $5/2+3/2 = 4 \neq 1$.
            \item % 1b
                Clearly sketch and label the solution curve and direction field. 
                \begin{center}
                    \includegraphics[scale=0.5]{figure.png}
                \end{center}
            \item % 1c
                What is the interval of validity for the function $y$ given the initial value?
                The function is defined only when the follwing is true: 
                    $$13/4 + x^3-e^x>0$$ 
                Using a computer we find that $x \in (-1.44, 4.63)$.
        \end{enumerate}
    \item % 2 
        Second question goes here
        \begin{enumerate}
            \item % 2a
                two a goes here
            \item % 2b 
                two b goes here
        \end{enumerate}
    \item % 3
        Third question here
    \item % 4 
        Fourth question goes here
\end{enumerate}




\end{document}

The role of technology in my day to day life

Technology plays an interesting role in my life.  Professionally, it is central to what I do on a day-to-day basis. I'm a full time online math instructor for AP & College level math at JHU CTY Online, so virtual meetings, email, graphing software, etc. are my bread & butter. Communication of complex mathematical ideas is what I do, and I make sure to take advantage of and master all relevant tools.  Without doing so, I'd be out of a job!

Outside of work however, I purposefully keep my life as technology free as possible. 

I use a laptop that I purchased used in 2007 as my main computing device, largely for checking email, writing blogs, and following shows like Adventure Time & Community. My phone is only capable of sending and receiving phone calls.  No texting, no internet, it takes incredibly tiny pictures (480 x 640 pixels, which is just under 1/3rd of a megapixel).  I don't own a television, and my entertainment system is the same CD Player/alarm clock radio I've had since the last 90s. 

Does anyone else purposefully keep themselves tech-free in their lives outside of work? 

Just what exactly IS pre-calculus?

Different schools denote pre-calculus by many different names.  Any combinations of algebra I, II, III (Yes, Alg III), trig, or just "pre-calc" are considered "pre-calculus" depending on which school one is dealing with.  To each combination, one can add an "honors" prefix or a "with trig" suffix.

In deciding if a student is indeed ready to take calculus, I look at the topics covered in the class(es) they've taken in the past year.  If they match the list below, then I consider the student more or less ready to go and needing minimal remediation. It's worked out pretty well so far.

As far as I can see "Pre-Calculus" is the algebra and geometry of three main kinds of functions:

1. polynomials
2. exponential & logarithmic
3. trigonometric 

Students should be able to:

• recognize domain and range over bounded or unbounded intervals
• find x & y intercepts 
• understand scaling and translation f(x) vs a*f(b*(x - h)) + k
• find end behavior as x goes to +/- infinity
• find horizontal & vertical asymptotes
• understand function combination & composition
• understand the concept of an inverse and how it relates to domain & range, graphically & algebraically
• hand-sketch a given function and clearly highlight its important features
• evaluate functions at specific points
• algebraically manipulate & simplify trig identities, log rules, and factoring, cancelling, and collecting like terms
• know the entire unit circle and the trig sum & difference formulas
• use graphing software to dynamically visualize all of the above
• understand how each kind of function can be used to model real-world situations
• unambiguously communicate their thought process in their written solutions (or statements) of problems

I feel that students who enter my AP Calc class with these skills are well-prepared and tend to enjoy the course more because they are better able to see the calculus concepts through the "pre-calculus" algebraic manipulations, graphing, etc. 

What are your thoughts? Am I missing something?  Am I asking for too much? 

Khan Academy is not the only game in town for math videos.

This isn't a blog about bashing Khan Academy.  Khan Academy's math videos are good, but they are not the best. I mainly focus on math videos which are superior to Khan's.

Khan's analytics are the best. No doubt. Nobody comes close, at least as far as I know. When you have programming luminaries such as John Resig (creator of jQuery) on your team, the results are going to be outstanding.  The Khan Academy team is small, lean, efficient, and full of energy. The analytic tools available at Khan Academy are the best, hands down, bar none.

Khan Academy is not the only game in town for massive online problem sets, however. Try the Temple COW. http://www.math.temple.edu/~cow/ for problems in calc 1, 2, 3, linear algebra, number theory, and even abstract algebra.

I'm going to restrict myself to math videos for this blog post. All of the videos below have a few things in common:

1. Insight into the meaning of the mathematics. (not just rote problem-solving techniques)
2. Clearly chosen examples and
3. Well explained, nearly flawless delivery of any algebraic steps involved.

Here we go...

Math TV http://www.mathtv.com/videos_by_topic
Mr. McKeague is a lifelong educator who is one of my role models for pedagogy and professionalism. He has deep wisdom into teaching and life and he knows how to prepare and present sharply focused lessons that are approachable for students of all ages.  His website focuses largely on middle and secondary school math (number sense, algebra, trig, calculus). 

1. Advice for new teachers (good advice for any professional, really)
2. Following instructions (great for students!)

Midnight Tutors http://www.midnighttutor.com/
These guys were making online educational videos before Youtube was popular.  Unfortunately, they seemed to have stopped, but virtually all topics in AP Calculus are covered.  The main lecturer is professional educator and researcher in the sciences (rocket science, specifically) who has deep insight into the real life applicability of the math.  He is passionate, chooses clear illustrative examples, and does more than walk a student through a "bag of tricks" -- he encourages a way of thinking about mathematics which is rarely seen elsewhere.

1. Study tips -- applicable for all students
2. "Was this integral designed to torture calc students, or does it have meaning?"

MIT OCW (math superstars)
As you know, MIT has been producing free online videos (and readings, and java applets, etc.) in a variety of topics since around 2002.  Most of the video lectures are around 90 minutes in length, and not all of them are exactly the most engaging videos ever produced... but there are some gems out there!  Here are just a few.

Joel Lewis
All of his videos have extremely well-chosen examples, encourages students to try the problem on their own before revealing the solution, and gives plenty of intuition and interpretation of the results to highlight subtle points about the theorem or technique being used. Pretty much any video with Joel Lewis is gold.

1. Flux & Divergence Theorem

Herb Gross 
MIT filmed a calculus series back in the 70s with Dr. Herb Gross as the lecturer.  The lectures he gives are tremendously insightful, rigorous, yet still accessible. Herb makes sure to give ample explanation of the intuition behind each step of his reasoning and makes sure to constantly keep the "big picture" in mind as he goes through each lesson.  Also his accent reminds me of growing up in Bruk-Linn Noo Yawk. ;-p

1. The Multivariable chain rule and higher order mixed partial derivatives (I've never seen anyone explain it so clearly!)

Arthur Mattuk 

Arthur's writing (single variable calc, multivariable calc, diffeqs ) is just as good as his speaking. He uses clever metaphors to illustrate his examples and where such metaphors fail, he uses sterling clarity to choose examples to show where a theorem applies and where it may fail entirely.  He has a deep mathematical understanding of complex topics and their relationships between one another, as well as a great sense of humor.

1. Repeated Real Eigenvalues (Using the love between George Costanza and his wife Susan from Seinfeld to model & interpret a system of linear differential equations.)  

PatrickJMT (not with MIT) His Playlists

Patrick's math videos are usually short, to the point, feature a single, very clearly worked example, and start with a short summary of the theorem or property being used in the video.  His videos are great for illustrating example problems and a good strategy (for all videos, not just his) would be to pause and try to solve the problem before he does.  His videos are plentiful and thoughtfully composed. 

1. Infinite Series Review
2. Graphing a polar curve part 1, part 2